About Naoki Masuda

Affiliation etc.

Find me on

Github

Faculty Opinions

Interview by Society for Mathematical Biology

My main research interests are network science and mathematical biology, which overlap on top of each other. Let me briefly overview them and provide links to my representative work in each field.

Research interest 1: Network science

Networks are fun.

An introductory news article to my research

Some resources

My research topics in network science include the following:

temporal nerworks

Many networks are better considered to vary over time than being static. Or, specific times of contacts between two nodes may matter as much as the fact that the two nodes are directly connected by an edge. Such a temporal network view may change what has been known for static networks (e.g., how epidemics spread on networks). As network data with time stamps of events are increasingly available, we need develop algorithmic and statistical tools to analyze such temporal network data as well as mathematical/computational models to understand them including dynamical processes (e.g., contagion processes) on temporal networks.

Epidemic processes on networks

Understanding and intervening into epidemic processes occurring on networks is a major topic in network science due to its societal needs (including the case of information spreading in online media). I have been working on ``network epidemiology' both for temporal and static networks.

Research interest 2: Mathematical biology

There are a plenty of opportunities for mathematics to be used for biological and medical questions including data analysis. Reflecting the width of biology itself, mathematical biology is a broad discipline. I focus on the following topics in mathematical biology.

Behavioral biology Animal individuals in the same group interact with each other to be able to perform collective tasks such as nest selection in the case of ants. I am engaged in network analysis and mathematical modeling of such collective behavior of animals.

Energy landscape analysis

This is a method which we have been developing to understand multichannel dynamical data (i.e., multidimensional time series, which does not have to be obtained from a biological system) such as brain signals recorded at multiple regions of interest. The method aims to comprehend data as a dynamics among a small number of relatively stable states.

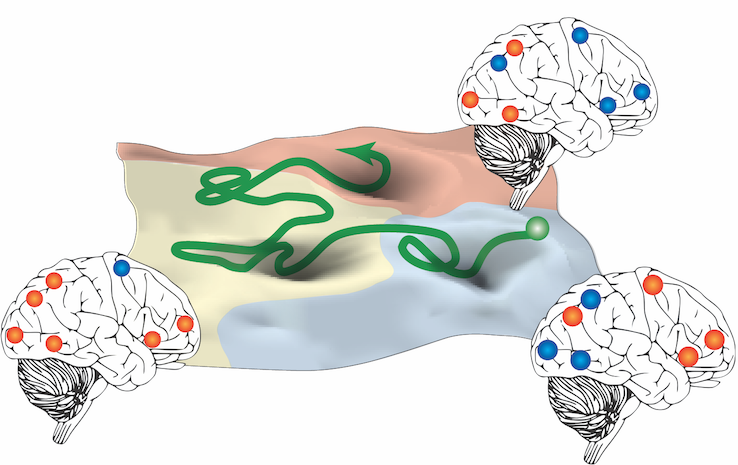Fig: Schematics of brain dynamics during bistable perception. Magenta: visual-area state. Blue: frontal-area state. Yellow: intermediate state. A colored circle represents activity of regions of interest in each of the three attractive basins (red: high, blue: low). The green curve represents brain dynamics.

Evolution of other mechanisms of cooperation

Evolutionary and other mechanisms of cooperation in soial dilemma situations have been a long-standing theme in mathematical biology. Nowadays, our understanding of this phenomenon is much enriched by behavioral experiments with humans and animals as well.

En español

Hablo español y me encanta viajar a España y países latinoamericanos. Me alegraré en tener estudiantes o posgrados que tengan interés en redes complejas o biología matemática (incluyendo redes en neurociencia). Si te interesa venir a mi centro de investigación, lee la información en ingrés arriba.

Aunque hablo inglés mucho mejor que español, no tengo problema en divertirme en español. Científicamente también, soy capaz de discutir en espanñol (pues, quizás estoy exagerando; solía hablar español mejor antes), que es lo que hago cuando veo investigadores latinos en conferencias. Mantengo la comunicación con investigadores latinos.

Aquí hay un articulo en español introduciendo nuestro trabajo [Speidel, Klemm, Eguíluz, Masuda. New Journal of Physics, 2016)]:
Importa cómo es la secuencia de las interacciones.
Revista Española de Física, Vol. 30, No. 4, 27-28 (2016).
No lo escribí yo...

Me he ubicado a los Estados Unidos en 2019. Espero que aquí hay más oportunidades de hablar castellano que antes.